Lysario – by Panagiotis Chatzichrisafis
"ούτω γάρ ειδέναι το σύνθετον υπολαμβάνομεν, όταν ειδώμεν εκ τίνων και πόσων εστίν …"
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 35 exercise 2.18
Author: Panagiotis22 Aug
In [1, p. 35 exercise 2.18] we are asked to prove that the inverse of a complex matrix 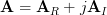 may be found by first inverting
may be found by first inverting
to yield
and then letting .
read the conclusion >
.
read the conclusion >
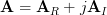 may be found by first inverting
may be found by first inverting
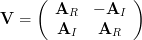 | (1) | ||
to yield
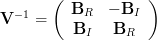 | (2) | ||
and then letting
 .
read the conclusion >
.
read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 35 exercise 2.17
Author: Panagiotis16 Aug
In [1, p. 35 exercise 2.17] we are asked to verify the alternative expression [1, p. 33 (2.77)] for a hermitian function.
read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 35 exercise 2.16
Author: Panagiotis12 Aug
In [1, p. 35 exercise 2.16] we are asked to verify the formulas given for the complex gradient of a hermitian and a linear form [1, p. 31 (2.70)]. To do so, we are instructed to decompose the matrices and vectors into their real and imaginary parts as
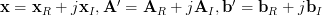 read the conclusion >
read the conclusion >
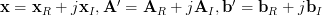 read the conclusion >
read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 35 exercise 2.15
Author: Panagiotis25 Jun
In [1, p. 35 exercise 2.15] we are asked to verify the formulas given for the gradient of a quadratic and linear form [1, p. 31 (2.61)].
The corresponding formulas are
and
where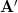 is a symmetric
is a symmetric 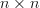 matrix with elements
matrix with elements 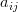 and
and 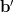 is a real
is a real 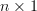 vector with elements
vector with elements 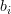 and
and 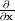 denotes the gradient of a real function in respect to
denotes the gradient of a real function in respect to
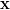 .
read the conclusion >
.
read the conclusion >
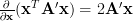 | (1) | ||
and
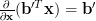 | (2) | ||
where
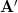 is a symmetric
is a symmetric 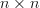 matrix with elements
matrix with elements 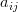 and
and 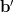 is a real
is a real 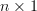 vector with elements
vector with elements 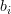 and
and 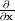 denotes the gradient of a real function in respect to
denotes the gradient of a real function in respect to
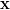 .
read the conclusion >
.
read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 35 exercise 2.14
Author: Panagiotis28 Mai
In [1, p. 35 exercise 2.14] we are asked to consider the solution to the set of linear equations
where is a
is a 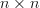 hermitian matrix given by:
hermitian matrix given by:
and is a complex
is a complex 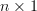 vector given by
vector given by
The complex vectors are defined in [1, p.22, (2.27)]. Furthermore we are asked to assumed that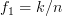 ,
, 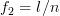 for
for 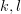 are distinct integers in the range
are distinct integers in the range ![[-n/2,n/2-1]](https://lysario.de/wp-content/cache/tex_24916734a0a5230271c84429761581eb.png) for
for  even and
even and ![[-(n-1)/2,(n-1)/2]](https://lysario.de/wp-content/cache/tex_b9ee4eee41d27a8e5eea256f738e00e2.png) for
for  odd.
odd. 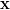 is defined to be a
is defined to be a 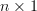 vector.
It is requested to show that
vector.
It is requested to show that  is a singular matrix (assuming
is a singular matrix (assuming 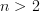 ) and that there are infinite number of solutions.
A further task is to find the general solution and also the minimum norm solution of the set of linear equations. The hint provided by the exercise is to note that
) and that there are infinite number of solutions.
A further task is to find the general solution and also the minimum norm solution of the set of linear equations. The hint provided by the exercise is to note that 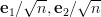 are eigenvectors of
are eigenvectors of  with nonzero eigenvalues and then to assume a solution of the form
with nonzero eigenvalues and then to assume a solution of the form
where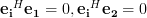 for
for 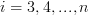 and solve for
and solve for 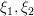 .
read the conclusion >
.
read the conclusion >
 | (1) | ||
where
 is a
is a 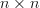 hermitian matrix given by:
hermitian matrix given by:
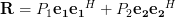 | (2) | ||
and
 is a complex
is a complex 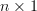 vector given by
vector given by
 | (3) | ||
The complex vectors are defined in [1, p.22, (2.27)]. Furthermore we are asked to assumed that
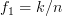 ,
, 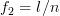 for
for 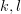 are distinct integers in the range
are distinct integers in the range ![[-n/2,n/2-1]](https://lysario.de/wp-content/cache/tex_24916734a0a5230271c84429761581eb.png) for
for  even and
even and ![[-(n-1)/2,(n-1)/2]](https://lysario.de/wp-content/cache/tex_b9ee4eee41d27a8e5eea256f738e00e2.png) for
for  odd.
odd. 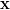 is defined to be a
is defined to be a 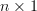 vector.
It is requested to show that
vector.
It is requested to show that  is a singular matrix (assuming
is a singular matrix (assuming 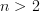 ) and that there are infinite number of solutions.
A further task is to find the general solution and also the minimum norm solution of the set of linear equations. The hint provided by the exercise is to note that
) and that there are infinite number of solutions.
A further task is to find the general solution and also the minimum norm solution of the set of linear equations. The hint provided by the exercise is to note that 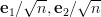 are eigenvectors of
are eigenvectors of  with nonzero eigenvalues and then to assume a solution of the form
with nonzero eigenvalues and then to assume a solution of the form
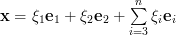 | (4) | ||
where
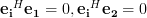 for
for 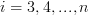 and solve for
and solve for 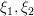 .
read the conclusion >
.
read the conclusion >