Lysario – by Panagiotis Chatzichrisafis
"ούτω γάρ ειδέναι το σύνθετον υπολαμβάνομεν, όταν ειδώμεν εκ τίνων και πόσων εστίν …"
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 61 exercise 3.5
Author: Panagiotis11 Jan
In [1, p. 61 exercise 3.5] we are asked to show that for the conditions of Problem [1, p. 60, exercise 3.4] the CR bound is
and further to give a statement about the efficiency of the sample mean estimator. read the conclusion >
 | |||
and further to give a statement about the efficiency of the sample mean estimator. read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 60 exercise 3.4
Author: Panagiotis28 Dez
In [1, p. 60 exercise 3.4] we are asked to prove that the estimate
is an unbiased estimator, given![\left\{x[0],x[1],...,x[N]\right\}](https://lysario.de/wp-content/cache/tex_1cc48dbb4a4db05229880d4eafd1756b.png)
are independent and identically distributed according to a 
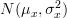distribution. Furthermore we are asked to also find the variance of the estimator.
read the conclusion >
![\hat{\mu}_x = \frac{1}{N}\sum\limits^{N-1}_{n=0}x[n]](https://lysario.de/wp-content/cache/tex_fdf7f47786a09a823706c0185f0d813a.png) | (1) | ||
is an unbiased estimator, given
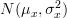are independent and identically distributed according to a distribution. Furthermore we are asked to also find the variance of the estimator.
read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 60 exercise 3.3
Author: Panagiotis4 Dez
In [1, p. 60 exercise 3.3] we are asked to prove that the complex multivariate Gaussian PDF reduces to the complex univariate Gaussian PDF if N=1.
read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 60 exercise 3.2
Author: Panagiotis8 Nov
In [1, p. 60 exercise 3.2] we are asked to proof by using the method of characteristic functions that the sum of squares of N independent and identically distributed N(0,1) random variables has a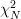 
distribution. read the conclusion >
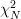distribution. read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 60 exercise 3.1
Author: Panagiotis10 Okt
In [1, p. 60 exercise 3.1] a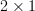 
real random vector 
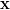is given , which is distributed according to a multivatiate Gaussian PDF with zero mean and covariance matrix:
We are asked to find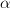
if 
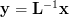where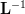 
is given by the relation:
so that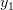
and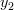 
are uncorrelated and hence independent. We are also asked to find the Cholesky decomposition of
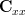which expresse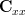s as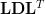 
, where 
is lower triangular with 1′s on the principal diagonal and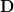 
is a diagonal matrix with positive diagonal elements. read the conclusion >
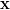real random vector is given , which is distributed according to a multivatiate Gaussian PDF with zero mean and covariance matrix:
![\mathbf{C}_{xx}=
\left[
\begin{array}{cc}
\sigma_{1}^2 & \sigma_{12} \\
\sigma_{21} & \sigma_{2}^2
\end{array}\right]](https://lysario.de/wp-content/cache/tex_372170732f621d352bb5f0949e11915d.png) | |||
We are asked to find
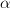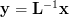if where 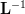is given by the relation: |  |  | |
| |  |
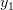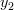so that
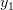and 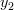are uncorrelated and hence independent. We are also asked to find the Cholesky decomposition of
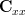which expresse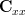s as 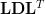, where is lower triangular with 1′s on the principal diagonal and 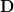is a diagonal matrix with positive diagonal elements. read the conclusion >