Lysario – by Panagiotis Chatzichrisafis
"ούτω γάρ ειδέναι το σύνθετον υπολαμβάνομεν, όταν ειδώμεν εκ τίνων και πόσων εστίν …"
Archive for April, 2011
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 61 exercise 3.7
Author: Panagiotis25 Apr
In [1, p. 61 exercise 3.7] we are asked to find the MLE of  and
and 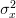 .
for the conditions of Problem [1, p. 60 exercise 3.4] (see also [2, solution of exercise 3.4]).
We are asked if the MLE of the parameters are asymptotically unbiased , efficient and Gaussianly distributed.
.
for the conditions of Problem [1, p. 60 exercise 3.4] (see also [2, solution of exercise 3.4]).
We are asked if the MLE of the parameters are asymptotically unbiased , efficient and Gaussianly distributed.
read the conclusion >
 and
and 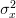 .
for the conditions of Problem [1, p. 60 exercise 3.4] (see also [2, solution of exercise 3.4]).
We are asked if the MLE of the parameters are asymptotically unbiased , efficient and Gaussianly distributed.
.
for the conditions of Problem [1, p. 60 exercise 3.4] (see also [2, solution of exercise 3.4]).
We are asked if the MLE of the parameters are asymptotically unbiased , efficient and Gaussianly distributed. read the conclusion >
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 61 exercise 3.6
Author: Panagiotis19 Apr
In [1, p. 61 exercise 3.6] we are asked to assume that the variance is to be estimated as well as the mean for the conditions of [1, p. 60 exercise 3.4] (see also [2, solution of exercise 3.4]) . We are asked to prove for the vector parameter ![\mathbf{\theta}=\left[\mu_x \; \sigma^2_x\right]^T](https://lysario.de/wp-content/cache/tex_b1d973967798bb8525bff936468f9211.png) , that the Fisher information matrix is
, that the Fisher information matrix is
Furthermore we are asked to find the CR bound and to determine if the sample mean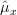 is efficient.
If additionaly the variance is to be estimated as
is efficient.
If additionaly the variance is to be estimated as
then we are asked to determine if this estimator is unbiased and efficient. Hint: We are instructed to use the result that
read the conclusion >
![\mathbf{\theta}=\left[\mu_x \; \sigma^2_x\right]^T](https://lysario.de/wp-content/cache/tex_b1d973967798bb8525bff936468f9211.png) , that the Fisher information matrix is
, that the Fisher information matrix is
![\mathbf{I}_{\theta}=\left[\begin{array}{cc} \frac{N}{\sigma^2_x} & 0 \\ 0 & \frac{N}{2\sigma^4_x} \end{array}\right]](https://lysario.de/wp-content/cache/tex_42789b24f24a6a32db03314ccac291c8.png) | |||
Furthermore we are asked to find the CR bound and to determine if the sample mean
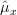 is efficient.
If additionaly the variance is to be estimated as
is efficient.
If additionaly the variance is to be estimated as
![\hat{\sigma}^2_x=\frac{1}{N-1}\sum\limits_{n=0}^{N-1}(x[n]-\hat{\mu}_x)^2](https://lysario.de/wp-content/cache/tex_13d6ef5f9b8f0b1d9832efd9b58af88b.png) | |||
then we are asked to determine if this estimator is unbiased and efficient. Hint: We are instructed to use the result that
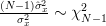 | |||
read the conclusion >