Lysario – by Panagiotis Chatzichrisafis
"ούτω γάρ ειδέναι το σύνθετον υπολαμβάνομεν, όταν ειδώμεν εκ τίνων και πόσων εστίν …"
Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”,p. 94 exercise 4.2
Author: Panagiotis24 Jun
In [1, p. 94 exercise 4.2] we are asked to consider the estimator
where
for the process of Problem 4.1. We are informed that this estimator may be viewed as an averaged periodogram. In this point of view the data record is sectioned into blocks (in this case, of length 1) and the periodograms for each block are averaged. We are asked to find the mean and variance of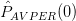 and compare the result to that obtained in [2].
and compare the result to that obtained in [2].
Solution: We note that according to the boundaries of problem 4.1 ( for which the process is white Gaussian with zero mean) we obtain the fact that the sum![U = \sum_{m=0}^{N-1}\frac{x^{2}[n]}{\sigma_{x}^{2}}](https://lysario.de/wp-content/cache/tex_17170bea01e2e71414bbc3c1720d276b.png) is distributed according to a
is distributed according to a 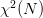 distribution [3, p. 682] with mean
distribution [3, p. 682] with mean 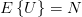 and variance
and variance 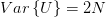 .
The average periodogram is obtained by
.
The average periodogram is obtained by 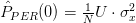 and thus the mean and the average is obtained by
and thus the mean and the average is obtained by
Thus taking the average periodogram of one sample section has no statistical advantage for the result of the estimator.
[1] Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”, Prentice Hall, ISBN: 0-13-598582-X.
[2] Chatzichrisafis: “Solution of exercise 4.1 from Kay’s Modern Spectral Estimation - Theory and Applications”.
[3] Granino A. Korn and Theresa M. Korn: “Mathematical Handbook for Scientists and Engineers”, Dover, ISBN: 978-0-486-41147-7.
 | (1) | ||
where
![\hat{P}_{PER}^{m}(0)= x^{2}[m]](https://lysario.de/wp-content/cache/tex_111f972a11d36418820c12baa2503f8a.png) | (2) | ||
for the process of Problem 4.1. We are informed that this estimator may be viewed as an averaged periodogram. In this point of view the data record is sectioned into blocks (in this case, of length 1) and the periodograms for each block are averaged. We are asked to find the mean and variance of
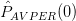 and compare the result to that obtained in [2].
and compare the result to that obtained in [2].
Solution: We note that according to the boundaries of problem 4.1 ( for which the process is white Gaussian with zero mean) we obtain the fact that the sum
![U = \sum_{m=0}^{N-1}\frac{x^{2}[n]}{\sigma_{x}^{2}}](https://lysario.de/wp-content/cache/tex_17170bea01e2e71414bbc3c1720d276b.png) is distributed according to a
is distributed according to a 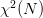 distribution [3, p. 682] with mean
distribution [3, p. 682] with mean 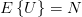 and variance
and variance 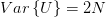 .
The average periodogram is obtained by
.
The average periodogram is obtained by 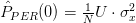 and thus the mean and the average is obtained by
and thus the mean and the average is obtained by
 | |||
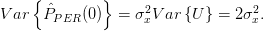 | |||
Thus taking the average periodogram of one sample section has no statistical advantage for the result of the estimator.
[1] Steven M. Kay: “Modern Spectral Estimation – Theory and Applications”, Prentice Hall, ISBN: 0-13-598582-X.
[2] Chatzichrisafis: “Solution of exercise 4.1 from Kay’s Modern Spectral Estimation - Theory and Applications”.
[3] Granino A. Korn and Theresa M. Korn: “Mathematical Handbook for Scientists and Engineers”, Dover, ISBN: 978-0-486-41147-7.
Leave a reply